Rearranging Formula ('Changing the Subject'): Explanation, Examples and Questions
Changing the subject of a formula is an algebraic skill which is required to solve equations and solve problems in applied mathematics.
Rearranging formula often includes changing the subject of the equation from one variable to another. You can change the subject of an equation by applying inverse operations.
In this article we show you step-by-step examples on how to rearrange equations so you can do this with ease.
What Does ‘Rearranging an Equation’ Mean?
Rearranging an equation means changing the form of the equation by performing operations such as addition and multiplication to both sides of the equation.
By doing the same to both sides of the equation, we make sure the equation remains balanced.
This allows us to change the subject of the equation or to rearrange the terms to simplify the equation.
What Does 'Change the Subject of the Equation’ Mean?
In the equation \( y = 5x + 7 \), y is the subject of the equation as the equation starts with 'y = '.
If we subtract 7 from both sides of the equation and then divide both sides by 5 we get:
\( x = \frac{y - 7}{5} \)
This is the same equation written in a different way so that the equation now starts with 'x = '
We have rearranged to make x the subject of the equation.
How to Rearrange Equations
To rearrange an equation, we need to look at which operations are being performed and do the opposite. Opposite operations are called inverse operations.
| Operation | Symbol | Inverse Operation | Symbol |
|---|---|---|---|
| Addition | + | Subtraction | − |
| Subtraction | − | Addition | + |
| Multiplication | × | Division | ÷ |
| Division | ÷ | Multiplication | × |
| Squaring | ² | Finding the square root | √ |
| Finding the square root | √ | Squaring | ² |
| Cubing | ³ | Finding the cube root | ∛ |
| Finding the cube root | ∛ | Cubing | ³ |
Step-by-Step Instructions
- Identify which variable you need to make the subject.
- Think about the order of operations being performed on this variable.
- Perform the inverse operations in reverse order to both sides of the equation until this variable is isolated.
If that sounds confusing, don't worry! We have plenty of examples.
Rearranging One Step Equations: Examples
Example 1
Question: Rearrange x = b + 5 to make b the subject.
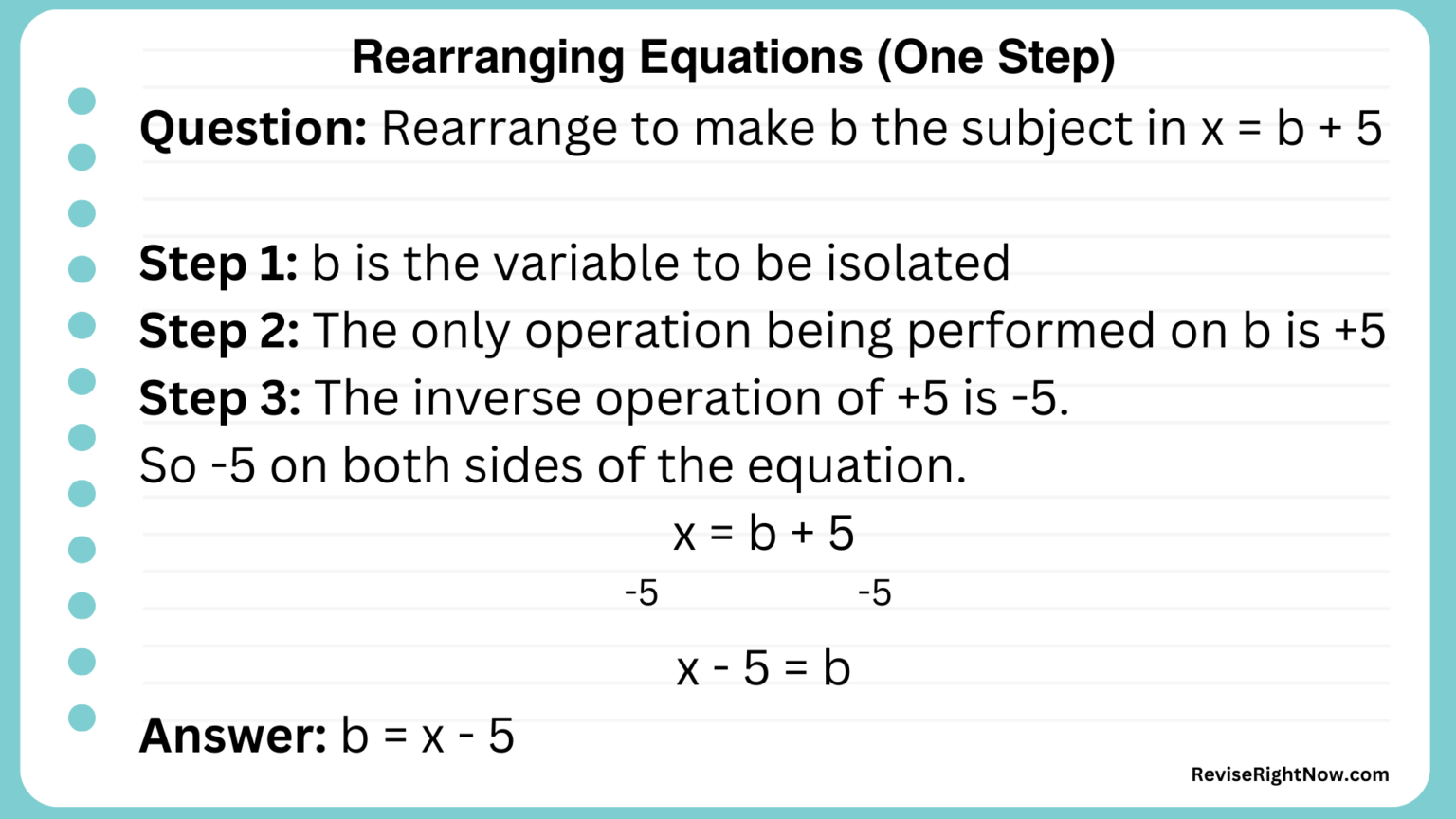
In this example, we are adding 5 to b. The opposite of adding 5 is subtracting 5 so we subtract 5 from both sides of the equation.
Answer: b = x - 5
Example 2
Question: Rearrange y = x ÷ 7 to make x the subject.
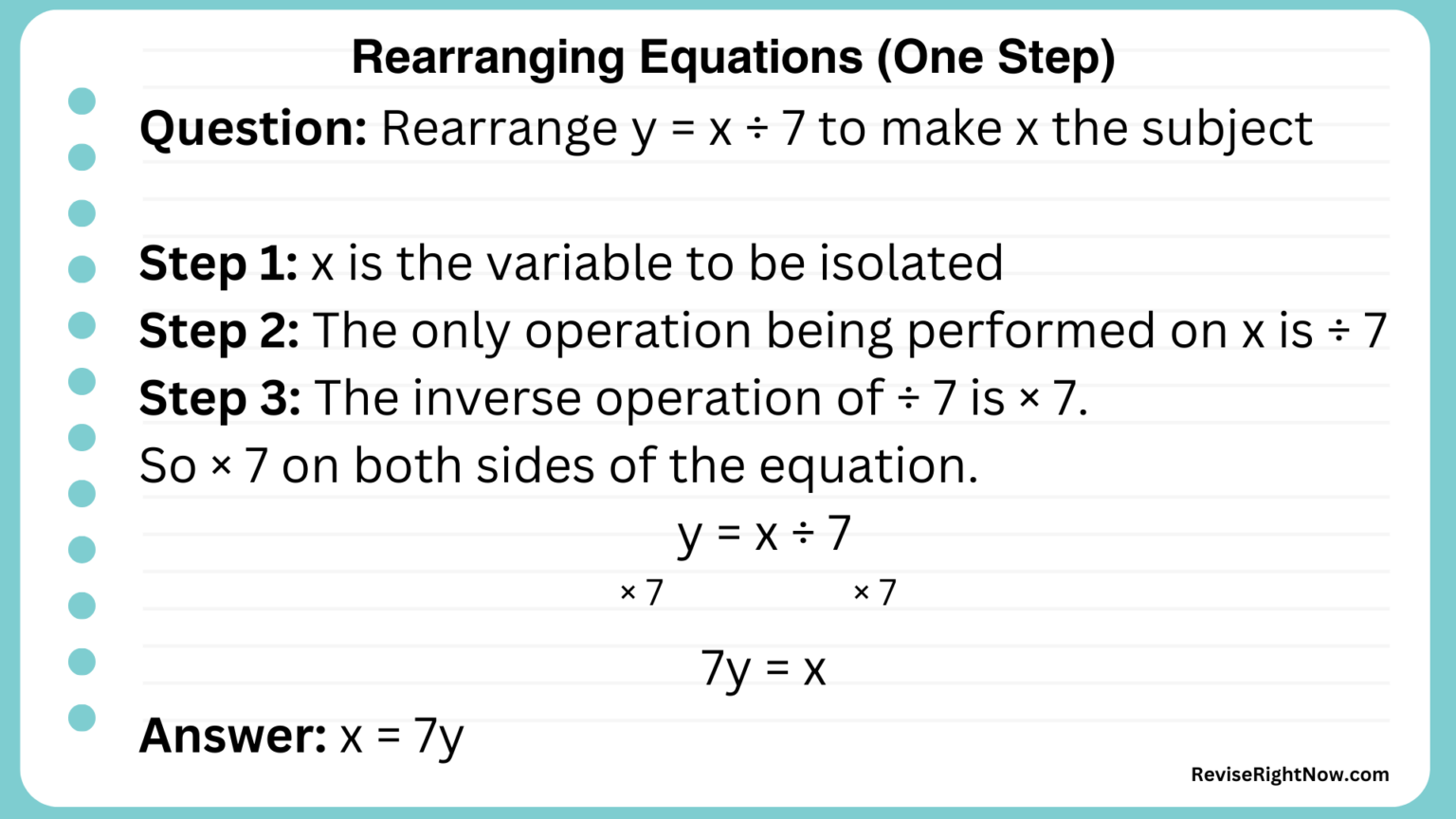
In this example, the operation being performed on x was ÷ 7 so we simply × 7 on both sides of the equation.
Answer: x = 7y
More on rearranging one step equations - coming soon.
Rearranging Two Step Equations: Examples
Example 3
Question: Rearrange y = 5x + 8 to make x the subject.
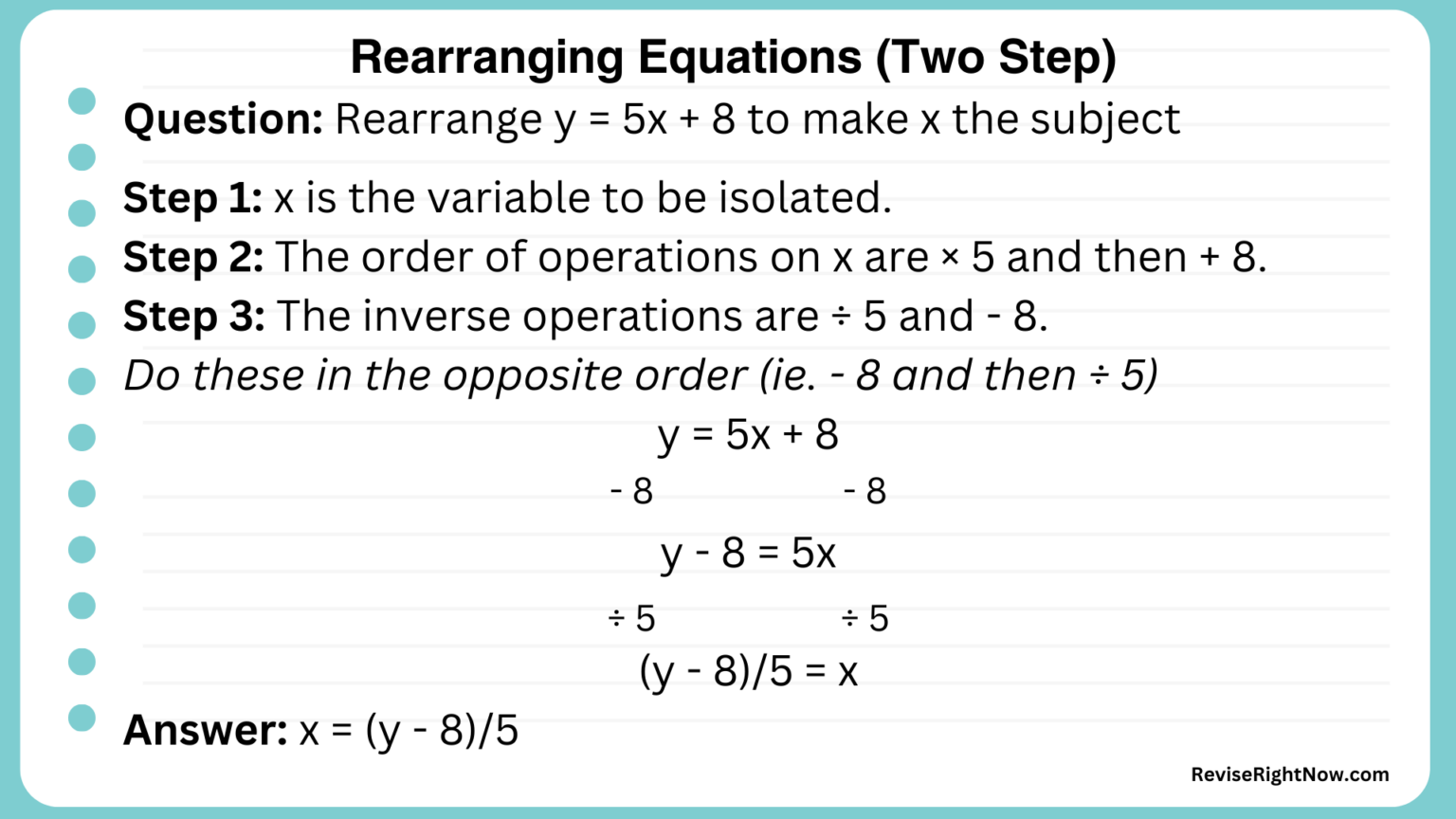
In this example, the operations on x are multiply by 5 and then add 8.
To make x the subject we need to do the inverse operations in the reverse order. Ie. subtract 8 and then divide by 5.
Answer: \( x = \frac{y - 8}{5} \)
Example 4
Question: Rearrange \( a = \sqrt{b} - 2 \) to make b the subject.
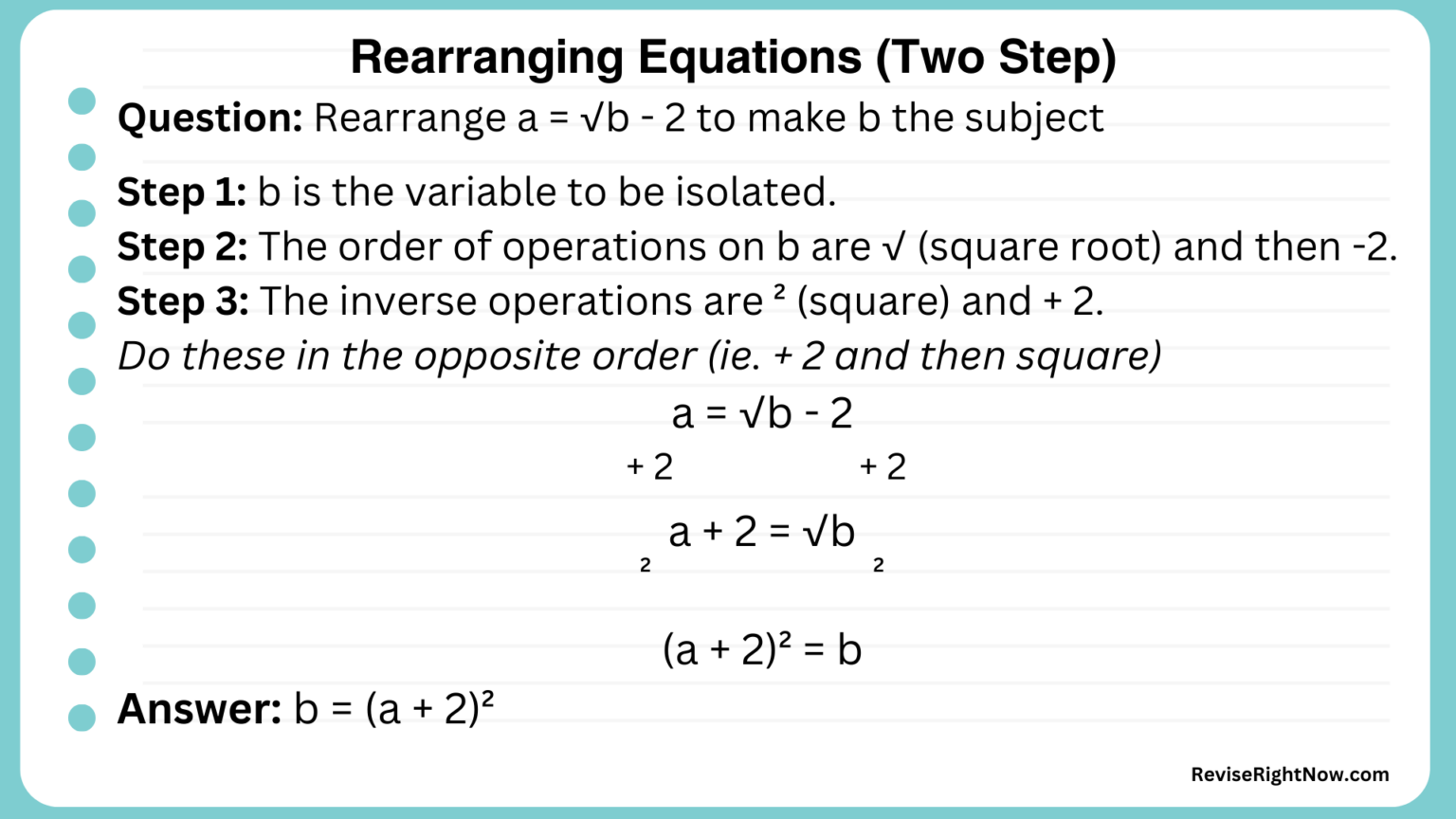
In this example, the operations on b are square root and then subtract 2.
To make b the subject, we need to do the inverse operations in the reverse order. Ie. plus 2 and then square.
Answer: \( b = (a + 2)^2 \)
More on rearranging two step equations - coming soon.
Rearranging Multi-Step Equations
Multi-step equations involve several operations. The method for solving them remains the same - by applying inverse operations in the reverse order.
How to Rearrange an Equation or Formula where the Subject Appears Twice
Sometimes the subject of the equation can appear twice. In this case you need to isolate the terms involving the subject on one side of the equation and factorise.
This video shows you how to do that.
Exam Practice Questions
Ready to practise some past paper questions? Choose your syllabus: