Rationalising the Denominator (with Examples and Practice Questions)
Rationalising the denominator is useful because it helps us simplify fractions with surds, making them easier to work with. By removing surds from the denominator, it allows for easier manipulation of algebraic expressions.
What Does Rationalising the Denominator Mean?
Rationalising the denominator means rewriting a fraction with an irrational denominator as an equivalent fraction with a rational denominator.
A rational number is one that can be written as a fraction. Surds such as \( \sqrt{7} \) and \( \sqrt{11} \) are irrational numbers.
When we rationalise a denominator, we want to replace the surd with a whole number.
For example, \( \frac{2}{\sqrt{3}} \) can be rewritten as \( \frac{2\sqrt{3}}{3} \).
How to Rationalise the Denominator
Rationalising the Denominator Method
If you multiply the numerator and denominator by the same number, you get an equivalent fraction.
For example:
\( \frac{3}{5} = \frac{3 \times 2}{5 \times 2} = \frac{6}{10} \)
When the denominator has only one term, we can rationalise the denominator by multiplying the numerator and denominator by the same surd.
Eg. \( \frac{2}{\sqrt{5}} = \frac{2 \times \sqrt{5}}{\sqrt{5} \times \sqrt{5}} = \frac{2\sqrt{5}}{5} \)
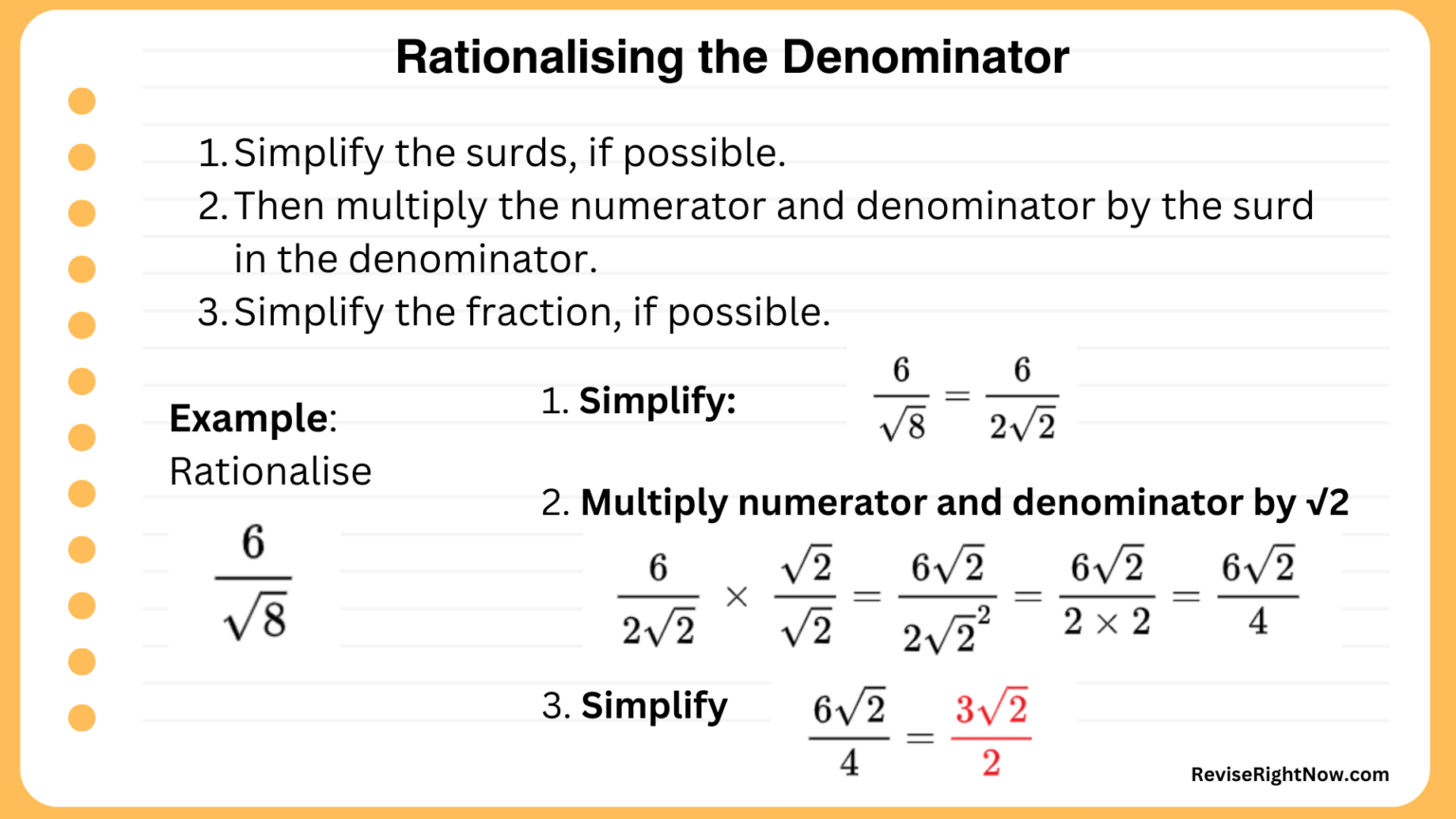
Step-by-Step Method
 /
/
- Simplify the surds first, if possible.
- Multiply the numerator and denominator by the surd in the denominator.
- Simplify the fraction, if possible.
Examples
Example 1: Rationalise \( \frac{6}{\sqrt{3}} \)
\( \frac{6}{\sqrt{3}} = \frac{6 \times \sqrt{3}}{\sqrt{3} \times \sqrt{3}} = \frac{6\sqrt{3}}{3} = 2\sqrt{3} \)
Example 2: Rationalise \( \frac{8}{\sqrt{24}} \)
Simplify first: \( \sqrt{24} = \sqrt{4 \times 6} = 2\sqrt{6} \).
Then \( \frac{8}{\sqrt{24}} = \frac{8}{2\sqrt{6}} = \frac{8\sqrt{6}}{12} = \frac{2\sqrt{6}}{3} \).
Example 3: Rationalise \( \frac{\sqrt{3}}{4\sqrt{5}} \)
\( \frac{\sqrt{3}}{4\sqrt{5}} = \frac{\sqrt{3} \times \sqrt{5}}{4\sqrt{5} \times \sqrt{5}} = \frac{\sqrt{15}}{20} \).
Rationalising the Denominator (with 2 Terms)
When the denominator has 2 terms, we use a conjugate. The conjugate is formed by changing the sign between two terms.
For example, the conjugate of \( 3 + \sqrt{2} \) is \( 3 - \sqrt{2} \).
How to Rationalise a Denominator with 2 Terms
- Simplify the surds, if possible.
- Multiply the numerator and denominator by the conjugate of the denominator.
- Simplify the fraction, if possible.
Examples
Example 1: Rationalise \( \frac{2}{\sqrt{5} + 3} \)
Multiply numerator and denominator by \( \sqrt{5} - 3 \):
\( \frac{2}{\sqrt{5} + 3} = \frac{2(\sqrt{5} - 3)}{(\sqrt{5} + 3)(\sqrt{5} - 3)} = \frac{2\sqrt{5} - 6}{5 - 9} = \frac{-2\sqrt{5} + 6}{4} \).
Example 2: Rationalise \( \frac{8}{\sqrt{6} - 2} \)
Multiply numerator and denominator by \( \sqrt{6} + 2 \):
\( \frac{8}{\sqrt{6} - 2} = \frac{8(\sqrt{6} + 2)}{(\sqrt{6} - 2)(\sqrt{6} + 2)} = \frac{8\sqrt{6} + 16}{6 - 4} = 4\sqrt{6} + 8 \).