Adding and Subtracting Surds
Just like learning to add fractions and decimals, there are rules for adding and subtracting surds. Fortunately, the process is actually quite simple. If you know what a surd is and how to simplify surds, then you’re ready to learn how to add and subtract surds.
What is a Surd?
A surd is any root whose value is an irrational number. An irrational number cannot be written as a fraction. The digits after the decimal point continue forever and never repeat.
| Surd | Not a Surd |
|---|---|
| √2 = 1.414213562… | √4 = 2 |
| √13 = 3.605551275… | √81 = 9 |
| √27 = 5.196152423… | √0 = 0 |
| 3√3 = 1.44224957… | 3√1 = 1 |
| 3√21 = 2.758924176… | 3√27 = 3 |
| 4√20 = 2.114742527… | 4√81 = 3 |
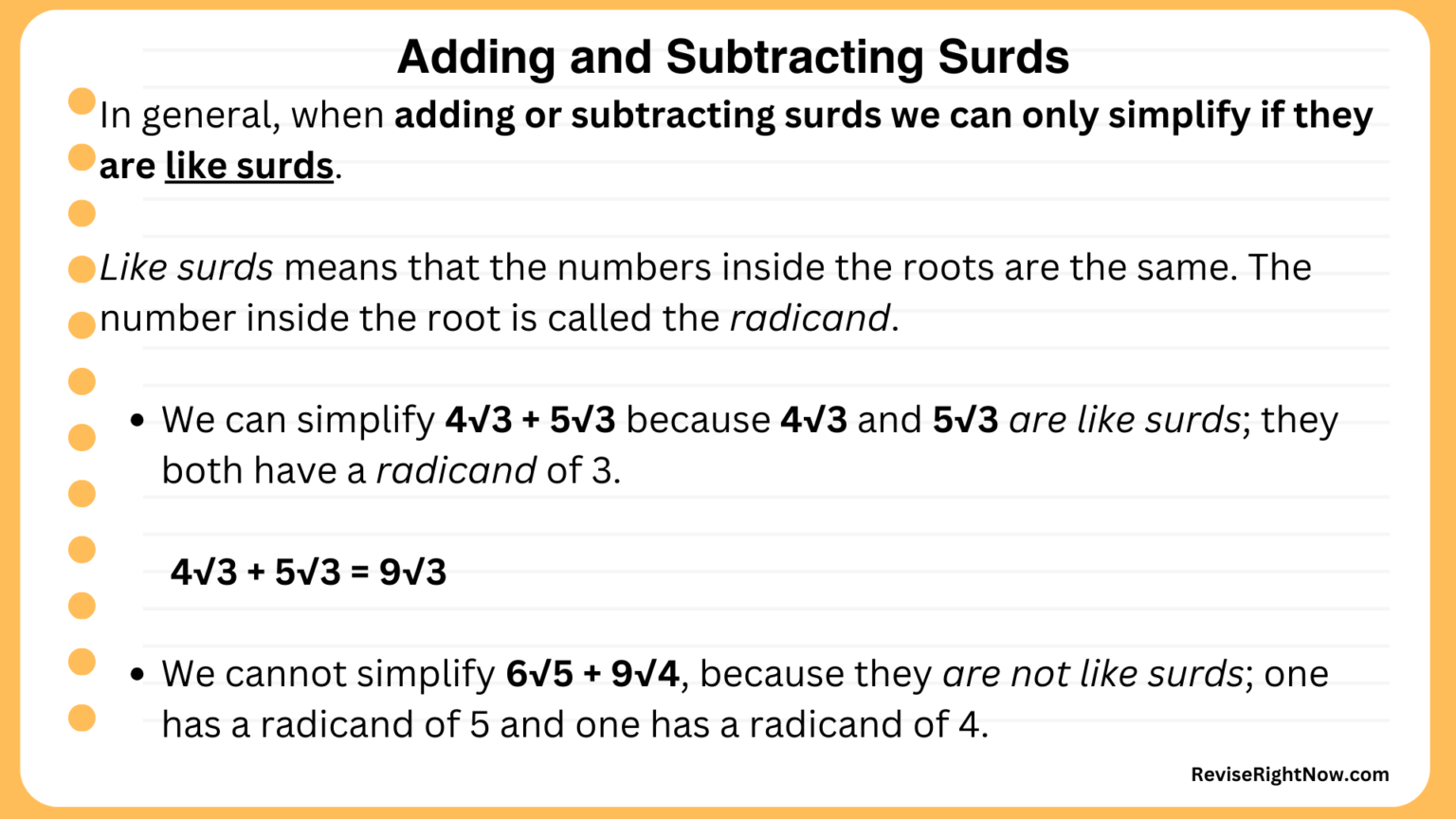
To see how to add and subtract surds, first consider this algebraic expression: 2x + 3x.
Since these are like terms, we can simplify: 2x + 3x = 5x. Similarly, if x = √7, then 2√7 + 3√7 = 5√7.
Like surds share the same radicand. For example, 6√5 + 9√5 simplifies to 15√5 because both have a radicand of 5, but 6√5 + 9√4
How to Add and Subtract Surds